Validating the Truth of Creation
Genesis 1:1 – “In the Beginning God Created the Heavens and the Earth”
Series 1 – Creation’s Code - Mathematics
Part 1 – Mandelbrot Equation – A glimpse into the mind of God
Introduction
The subject of Mathematics tends to bring on one of two responses.
- No problem, provided it is not too complicated and
- I don’t like maths for this reason xxxxxx.
However, whatever response the sight of the word ‘Mathematics’ elicited in you, rest assured you do not need to calculate any maths to be able to understand this beautiful evidence for God’s existence.
This article will endeavour to convey reasons for confidence that there really is a God, one who created all things, as opposed to us being here by blind chance as per the theory of Evolution.
So please continue on this examination with me, because it is truly stunning!
Mathematics
When we see a beautiful or captivating painting such as the Mona Lisa, we can appreciate it, and be in awe of its creator even though we could never aspire to paint in such a way. It is likewise with Mathematics, we may barely understand it, but we can still appreciate its beauty, for it truly is beautiful!
What is Mathematics?
- Mathematics is the study of the relationships between numbers.
What are numbers?
- They are best explained as a concept of quantity.
What are numerals then?
- Written numerals are not numbers, they are how we express the concept of numbers in written and visual form.
- They are merely representations of numbers.
Additionally, a key point to keep in mind is that all the laws of math are conceptual.
- A concept is something conceived in the mind.
Basis
We are all familiar with the concept of a “Set”. You may well have a set of playing cards, or a set of chess pieces or a set of Wine glasses.
Therefore, we can understand that the definition:
SET: = a collection of elements with a common defined property.
To illustrate, each individual playing card is an element of the entire set of cards, and likewise each individual chess piece is an element of the entire chess set. Additionally a wine glass is one of a set of glasses of a particular shape with properties designed to bring out the best from the wine, such as the smell, and the appearance.
Similarly, in maths, a set of numbers is a collection of numbers with a particular property or properties that define that set but may not be in another collection.
For example, take the following numbers: 0, -2, 1, 2, -1, 3, -3, -½, ½.
Of those numbers the following belong to
- Negative Set: {-2, -1, -3, -½}
- Positive Set: {1, 2, 3, ½}
- Fractions Set: {-½, ½}
- Whole Number Positive: {1, 2, 3}
And so forth.
One such set is the Mandelbrot set:
This is the set of all numbers (c) for which the formula Zn2 + c = Zn+1 and Zn remains small.
Establishing numbers part of the Mandelbrot set
As an example, to check if the number 1 is part of the Mandelbrot set:
If c = 1 then start with Zn = 0.
Replacing these numbers in this formula we get:
(Z)02 + (c)1 = 1. Therefore Zn = 0 and 1.
Next taking the result of 1, setting Z=1 we get:
(Z)12+ (c)1=2.
Next taking the result of 2, setting Z=2 we get:
22+1 = 5
Next taking the result of 5, setting Z=5 we get:
52+1 = 26
Next taking the result of 26, setting Z=26 we get:
262+1 = 677
Therefore Zn=0, 1, 2, 5, 26, 677, …
We can therefore see that the value of c = 1 is not part of the Mandelbrot set as the number does not stay small, in fact very quickly it has become 677.
So, is c = -1 part of the Mandelbrot set?
The short answer is yes, as following the same steps as followed above we get the following sequence of numbers.
Starting again with Zn = 0. Replacing these numbers in this formula we get:
(Z) 02 (c)-1 = -1. Therefore Zn = -1.
Next taking the result of -1, setting Z=-1 we get:
-12 -1 = 0.
Next taking the result of 0, setting Z=0 we get:
02-1 = -1
Next taking the result of -1, setting Z=-1 we get:
-12 -1 = 0.
Next taking the result of 0, setting Z=0 we get:
02-1 = -1
The result is that Zn= 0, -1, 0, -1, 0, -1, 0, -1, ….
Therefore we can see that c= -1 is part of the Mandelbrot set as it always stays small.
There is one more concept we need to discuss as background before being able to see the beauty.
The Mandelbrot set also contains ‘imaginary’ numbers.
- The square of an ‘imaginary number’ is a negative number.
- Such as in i2= -1 where i is the imaginary number.
To visualize them think of the horizontal x axis of a graph having the Negative numbers through zero to Positive numbers. Then the Y axis going vertically from -i, - ½i through zero (the cross point of the two axis) and upwards to ½i and i.
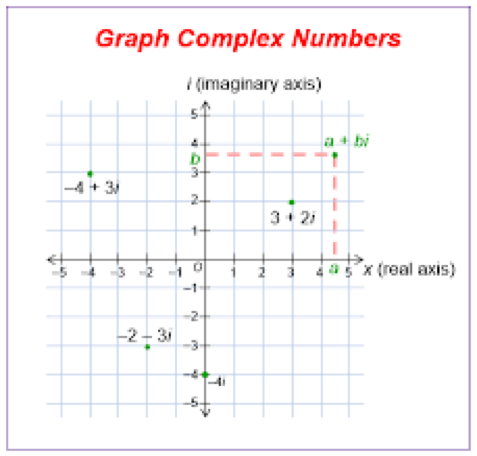
Diagram 1: Showing imaginary numbersOther numbers in the Mandelbrot set are 0, -1, -2, ¼, whereas 1, -3, ½ are not. More numbers in this set include i, -i, ½i , - ½I, but 2i, -2i are not.
That is the end of all the complicated maths.
Now this is where it gets really interesting!
The Results of this formula
As you can imagine to calculate and then plot all the valid and invalid values by hand would take a very long time.
However computers can be put to very good use to calculate 100’s of thousands, even millions of values and then to plot the results of this formula visually on a graph.
To easily identify by eye the valid points are marked in black, the invalid points are marked in red, and the points that are very close, but not quite valid are marked in yellow.
If we run a computer program to do that, we get the following result shown below.
(You can try it for yourself with various online programs such as the following:
- http://math.hws.edu/eck/js/mandelbrot/MB.html
- https://sciencedemos.org.uk/mandelbrot.php
- http://www.jakebakermaths.org.uk/maths/mandelbrot/canvasmandelbrotv12.html
- http://davidbau.com/mandelbrot/
- https://fractalfoundation.org/resources/fractal-software/
- https://www.youtube.com/watch?v=PD2XgQOyCCk
)

Diagram 2 : Result of Mapping the Mandelbrot equation
Discovery 1
We start counting the yellow branches on the large black balls on the large black kidney like shape.
On the top small black circle on top of the large black kidney shaped area we have 3 branches. If we move to the next smallest circle on the left, we find 5 branches.
The next largest to the left has 7, and so forth, 9, 11, 13, etc, all the odd numbers to odd infinity.
[caption id="attachment_16647" align="alignnone" width="660"]
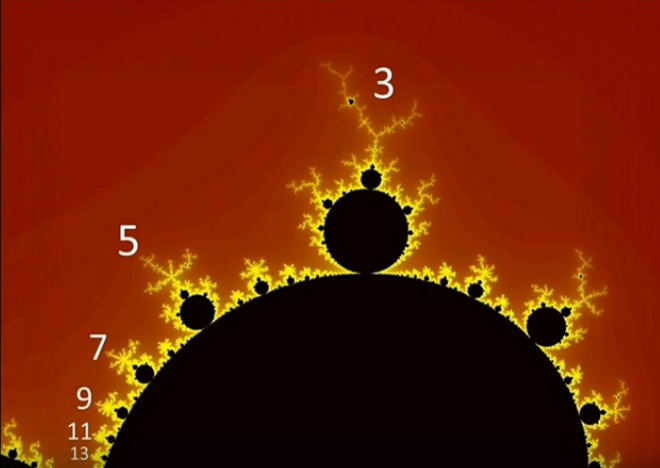 Diagram 3: Branches[/caption]
Diagram 3: Branches[/caption]Discovery 2
Now, going to the right of the black kidney shape from the top it knows how to count. We get 4, 5, 6, 7, 8, 9, 10, and onwards as the count of branches on the top of the largest black balls.
Discovery 3
But we haven’t finished yet. Going to the left from the top, the largest black circle from the top between the 3 and 5 branch circles has 8 branches, the sum of the branches from the circles either side! And between 5 and 7 the smaller black circle has 12, and so forth.
The same sums are found going to the right. So, the largest ball between 3 and 4 has 7 branches, and between 4 and 5 has 9 branches and so on.
[caption id="attachment_16648" align="alignnone" width="1441"]
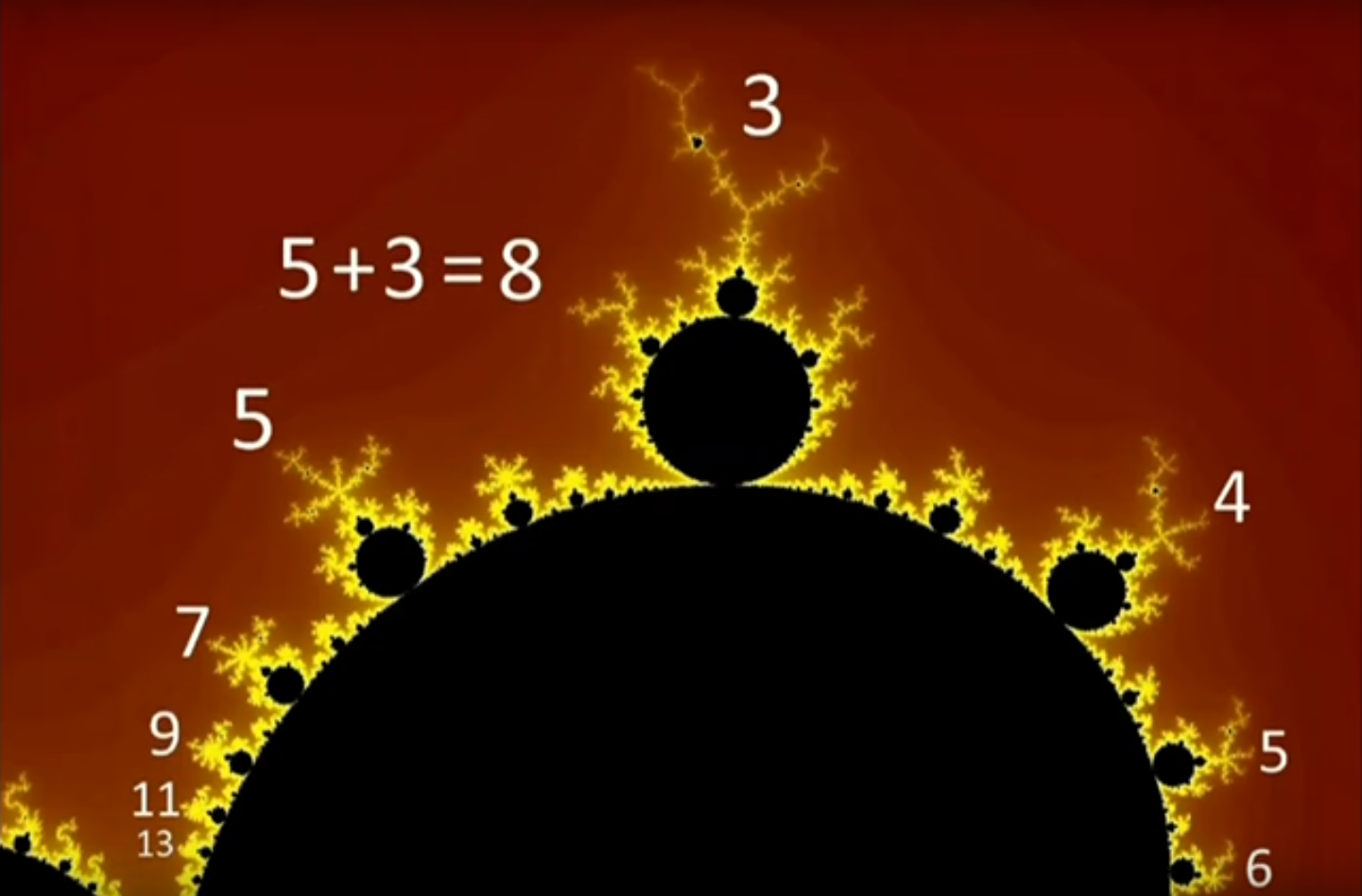 Diagram 4: Branches can do maths as well![/caption]
Diagram 4: Branches can do maths as well![/caption]Discovery 4
Furthermore, these shapes can be continuously magnified, and the same shapes will repeat.
[caption id="attachment_16649" align="alignnone" width="673"]
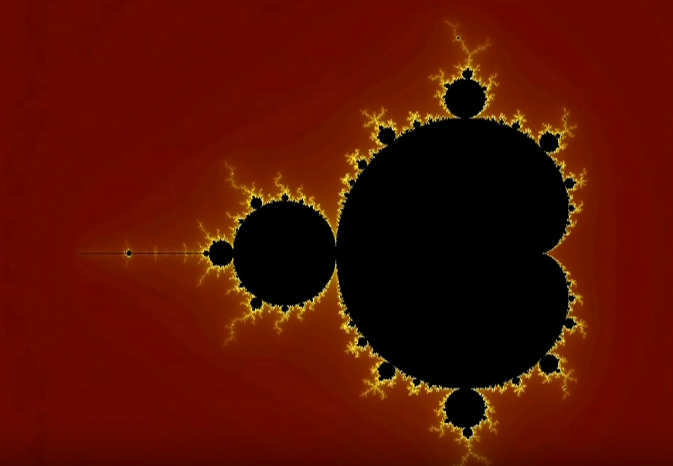 Diagram 5: Same pattern repeated infinitely[/caption]
Diagram 5: Same pattern repeated infinitely[/caption]The little black dot on the far left of the black line going to the left, if magnified is the same image as we see here. It is truly mind boggling.
Discovery 5
Between the larger heart shape and the attached black circle on the left is an area looking like Seahorse valley for the beautiful shapes seen there.
[caption id="attachment_16650" align="alignnone" width="625"]
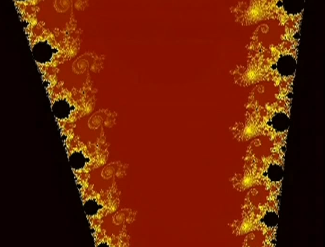 Diagram 6: Valley of the Seahorses![/caption]
Diagram 6: Valley of the Seahorses![/caption]Changing the red for blue and the yellow for white for easier contrast, when we zoom in closer, we see more beautiful patterns and more repeats of the basic pattern of the black kidney-shaped with an attached ball on the left.
[caption id="attachment_16651" align="alignnone" width="449"]
 Diagram 7: Seahorse in closeup[/caption]
Diagram 7: Seahorse in closeup[/caption]Zooming in on the bright white spot we see:
[caption id="attachment_16652" align="alignnone" width="674"]
 Diagram 8: Detail of Whitish whorl in centre of Seahorse[/caption]
Diagram 8: Detail of Whitish whorl in centre of Seahorse[/caption]And zooming further in even more on the centre spot we get the following:
[caption id="attachment_16653" align="alignnone" width="684"]
 Diagram 9: Extra Zoom in![/caption]
Diagram 9: Extra Zoom in![/caption]Zooming in yet more we find another of our basic shapes:
[caption id="attachment_16654" align="alignnone" width="674"]
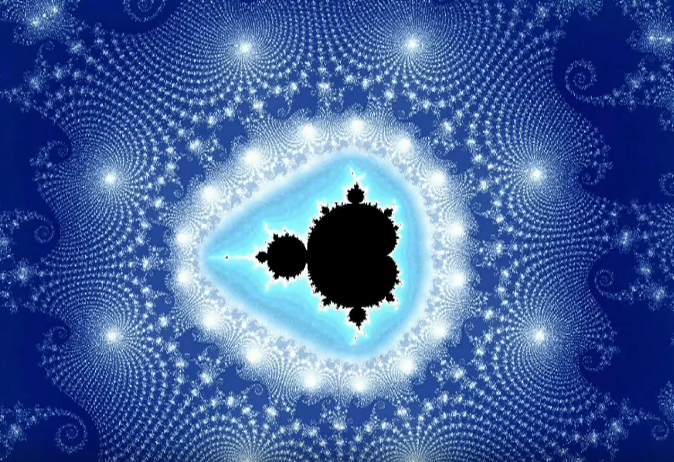 Diagram 10: Its that shape again[/caption]
Diagram 10: Its that shape again[/caption]If we zoom in on one of the whirls, we get the following:
[caption id="attachment_16655" align="alignnone" width="650"]
 Diagram 11: Spiralling In Control[/caption]
Diagram 11: Spiralling In Control[/caption]And at the centre of the whirl we get the following:
[caption id="attachment_16656" align="alignnone" width="692"]
 Diagram 12: Is it my eyes going in whirls too?[/caption]
Diagram 12: Is it my eyes going in whirls too?[/caption]Zooming in further on one of the two whirls we get the following two pictures which include yet another starting Mandelbrot kidney shape and ball.
[caption id="attachment_16657" align="alignnone" width="697"]
 Diagram 13: Just when you thought you had seen the last of that black shape![/caption]
Diagram 13: Just when you thought you had seen the last of that black shape![/caption][caption id="attachment_16658" align="alignnone" width="692"]
 Diagram 14: Yes, it is back again, surrounded by a different beautiful pattern[/caption]
Diagram 14: Yes, it is back again, surrounded by a different beautiful pattern[/caption]Discovery 6
Going back to our first picture of the Mandelbrot set and turning to the ‘valley’ on the right-hand side of the large heart shape and zooming in we see elephant-like shapes, which we will name Elephant valley.
[caption id="attachment_16659" align="alignnone" width="658"]
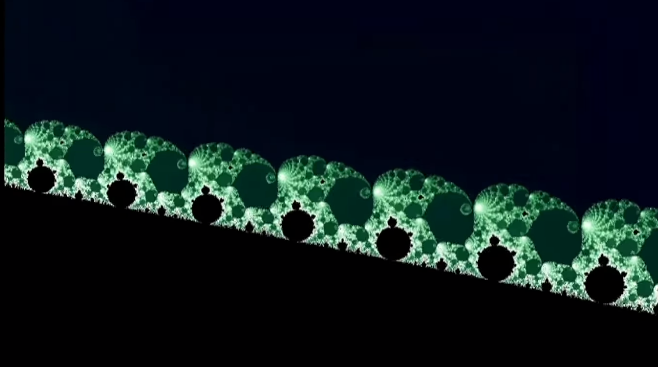 Diagram 15: Elephant Valley[/caption]
Diagram 15: Elephant Valley[/caption]As we zoom in, we get another set of beautiful but different repeating shapes as follows:
[caption id="attachment_16660" align="alignnone" width="648"]
 Diagram 16: Follow the Herd. Hup two, three, four, Elephant march.[/caption]
Diagram 16: Follow the Herd. Hup two, three, four, Elephant march.[/caption]We could go on and on.
Discovery 7
So, what causes the beauty in these Fractals from the Mandelbrot equation?
Yes, the computer may have applied a man-made colour scheme, but the patterns which the colours highlight are the result of the mathematical formula which has always existed. It cannot evolve, or change.
The beauty is intrinsic in the maths, as is the complexity.
Discovery 8
You may have noticed one particular word keeps on appearing. That word is “concept”.
- A concept is abstract in nature.
- A concept only exists in our minds.
Discovery 9
This raises the following questions in the minds of thinking persons.
Where do the laws of math come from?
- Being a concept, they can only come from another mind, which must be of higher intelligence than ours to be valid throughout the universe.
Did the laws of math evolve? If so, how could they?
- Abstract things cannot evolve as they are not physical.
Did people invent or create these laws of Maths?
- No, the Laws of mathematics existed before people.
Do they come from the universe?
- No, something of order could not come from random chance. The universe does not have a mind.
The only conclusion we can come to is that they had to come from the mind of a being far superior to man. The only being they could reasonably come from therefore has to be the creator of the universe, hence from God.
The laws of mathematics are:
- conceptual,
- universal,
- invariant,
- exception-less entities.
They could only come from God because:
- God’s thoughts are conceptual (Isaiah 55:9)
- God created the universe (Genesis 1:1)
- God does not change (Isaiah 43:10b)
- God knows all heavenly creation, nothing missing (Isaiah 40:26)
Conclusions
- In this brief examination of fractals and the Mandelbrot equation we have seen the beauty and order intrinsic in Mathematics and the design of the universe.
- This gives us a glimpse into the mind of God, which clearly contains order, beauty and infinite variety and is evidence for a far more intelligent mind than humans.
- It also shows his love in that he gave us the intelligence to be able to discover and (another concept!) appreciate these things.
Let us therefore display that concept of appreciation for what he has created and for him as the creator.
Acknowledgements:
With grateful thanks for the Inspiration given by YouTube video “The Secret Code of Creation” from the Origins Series by Cornerstone Television Network.
Fair Use: Some of the pictures used may be copyrighted material, the use of which has not always been authorized by the copyright owner. We are making such material available in our efforts to advance understanding of scientific and religious issues, etc. We believe this constitutes a fair use of any such copyrighted material as provided for in section 107 of the US Copyright Law. In accordance with Title 17 U.S.C. Section 107, the material on this site is made available without profit to those who express an interest in receiving and viewing the material for their own research and educational purposes. If you wish to use copyrighted material that go beyond fair use, you must obtain permission from the copyright owner.
Archived Comments
We have moved to the Disqus commenting system. To post a new comment, go to the bottom of this page.
Comment by Truth_seeker on 2020-01-22 05:12:39
Yes, I was always amazed how natural sciences (eg. physics, chemistry, biology, etc.) can be interpreted and expressed with mathematics. It does, indeed, seem part of a master plan.
Comment by Fani on 2020-01-22 12:41:23
Merci beaucoup Tadua
Je n'ai pas tout compris dans le développement mais j'ai bien compris la conclusion et j'ai été émerveillée par les diagrammes.
Les mathématiques alliées à la beauté.! Quelle merveille !
Nous connaissons si peu de choses ; combien les cieux et son trône doivent être grandioses et beaux !
Cette complexité, cet ordre, cette beauté renforcent notre foi en notre Dieu Tout Puissant.
Gloire à Lui !
Comment by Alithia on 2020-01-24 20:21:17
Beautiful presentation Tadua.
The universal language of the material universe is mathematics. One can rightly ask how is it that the universe and all things in it can be explained in this way? And how is it that we as material creatures have the ability to both apprehend and comprehend this language and use it to know our universe?
As rightly pointed out math is an abstract reality that evolution cannot account for. Materialism and naturalism has no explanation for these immaterial realities that transcend material realities.
One of the greatest mathematical minds in the history of mankind, Albert Einstein made the following remarks as follows;
"The most incomprehensible thing about the universe is that it is comprehensible. Nothing that I can do will change the structure of the universe".
“One must divide one’s time between politics and equations. But our equations are much more important to me, because politics is for the present, while our equations are for eternity.”
Love to all from Alithia.Reply by Alithia on 2020-01-26 03:46:54
Hi again, if permissible, another beautiful presentation in the link provided demonstrating how math is the universal language of the universe and can be explained this way. It gives the lie to evolution which claims life is but a chaotic and random chance process .
Where as life and everything in the universe is exquisitely precise and ordered like a well set out equation.
https://youtu.be/0K-t090uvL4
